Reading: Debt Policy in a Perfect World

Let us start the discussion about the relevance of debt policy in a very stylized environment. While this environment is far away from reality, it will allow us to derive some very important basic relations for the firm's financing policy. Later, we will then relax some of the assumptions to reach a more realistic environment. As we relax these assumptions, we will see how financing policy matters, and why. So please bear with us for a moment.

The world we assume has the following characteristics (among others):

• Symmetric information and homogeneous expectations: All market participants have the same information and they read that information the same way. So there are no disagreements about future cash flows or the riskiness of these cash flows.
• Given investment policy: The firm's investment policy is fixed. Put differently, the way the firm is financed does NOT affect its business activities. Factually, this implies that financing decisions leave the left side of the balance sheets (assets) unaffected and "simply" change the structure of the rights side of the balance sheet (debt and equity).
• No taxes: For the moment, we also assume that there are no taxes (at the corporate as well as the personal level). 
• No costs of financial distress
• No transaction costs
• Firms and investors borrow (and invest) at the same rate

To simplify the following numerical analysis, we also assume that the firm in question is a very simple firm:

• It earns perpetual cash flows. Put differently, the firm is expected to generate the same amount of cash each year forever. This assumption allows us to use simple valuation formulas (namely level perpetuities) and thereby dramatically simplify the exposition.
• Net investments (capital expenditures) equal depreciation charges. 
• The net working capital remains constant.

With the latter two assumptions, accounting earnings constitute cash flows (please refer to the module "Financial Planning" for a full-fledged discussion of the firm's cash flow statement). Specifically:

• The cash flow to shareholders (equity or residual cash flow) equals net income
• the free cash flow equals operating profit (EBIT) minus taxes, a profitability measure which is known as Net Operating Profit Less Adjusted Taxes (NOPLAT) or Earnings Before Interest After Taxes (EBIAT).

Finally, we assume that, once a firm has chosen its financing policy, it will hold on to it in perpetuity. As a result, debt outstanding will remain constant going forward, so that so that interest expenses are the only cash flows to debtholders.

Thanks to all these assumptions, we can skirt the complexities surrounding financial planning and valuation and, instead, use the following formulas to value the firm as well a its debt and equity:

Market value of debt = \( \frac{\text{Debt cash flow}}{\text{Cost of debt}} = \frac{\text{Interest payments}}{\text{Cost of debt}} \)

Market value of equity = \( \frac{\text{Equity cash flow}}{\text{Cost of equity}} = \frac{\text{Net income}}{\text{Cost of equity}}\)

Market value of firm = Market value of debt + Market value of equity.

In these equations:

• Cost of capital is the average return the providers of the respective source of capital (in our case debt and equity) expect to earn on their investment.
• Cost of equity is the average return the providers of equity (shareholders) expect to earn on their investment.
• Cost of debt is the average return the providers of debt expect to earn on their investment.

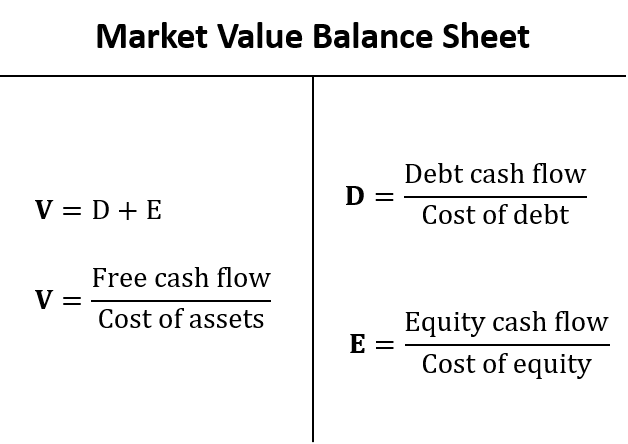
The following figure summarizes these valuation considerations with the so-called Market Value Balance Sheet. The overall value of the firm (denoted with the letter V) corresponds to the total value of the financial claims against the firm, i.e., the market value of debt (D) plus the market value of equity (E):

The theoretical stock price then corresponds to the equity value divided by the number of shares outstanding:

Stock price = \( \frac{\text{Equity value}}{\text{Number of shares}} \)

Alternatively, since our assumptions imply that net income equals equity cash flow, we can directly estimate the firm's theoretical stock price if we express net income on a per-share basis (earnings per share, EPS) and then capitalize EPS as a level perpetuity:
 

EPS = \( \frac{\text{Net income}}{\text{Number of shares}} \)

Stock price = \( \frac{\text{EPS}}{\text{Cost of equity}} \)

With these assumptions in place, we are now ready to study the relevance of a firm's debt policy with a numerical example.