Reading: Debt Policy in a Perfect World
2. An Unlevered Firm
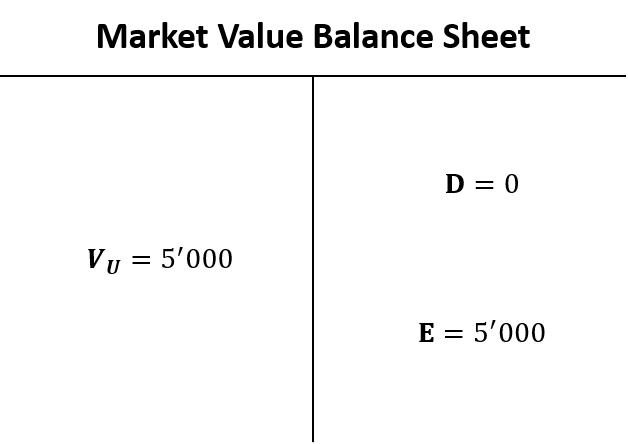
Let us first consider a fully equity-financed firm. Since the firm has no debt outstanding, its leverage ratio is zero, which is why such a firm is generally referred to as an unlevered firm. Let's assume the unlevered firm has 500 shares of common stock outstanding that currently trade at a price of $10 each. Consequently, the market value of the firm's equity (E) is 5'000 [= 10 × 500]. Since the firm is fully equity financed (no debt, D = 0), the overall value of the unlevered company (\(V_U\)) is also 5'000:
Three possible states of the world
We assume that there are three possible future states of the world that are unknown today and have equal probability: Recession, expected, boom. The following table shows the annual Earnings Before Interest and Taxes (EBIT) the firm expects to earn in the three scenarios:
| Recession | Normal | Boom | |
| EBIT | 300 | 600 | 900 |
In a first step, we investigate the returns that this firm promises to its investors as well as the relation between these returns and the valuation of the company. The next step will then be to change the firm's financing policy and to study how these changes affect the shareholders' returns and the valuation of the company.
The following table summarizes the key takeaways of the first step --- the company analysis under the current capital structure without any debt financing:
| Recession | Normal | Boom | Expected | |
| EBIT | 300 | 600 | 900 | 600 |
| - Interest expenses | 0 | 0 | 0 | 0.0 |
| Earnings before taxes | 300 | 600 | 900 | 600 |
| - Taxes | 0 | 0 | 0 | 0 |
| Net income | 300 | 600 | 900 | 600 |
| Debt cash flow | 0 | 0 | 0 | 0 |
| Equity cash flow | 300 | 600 | 900 | 600 |
| Return to total capital (%) | 6.0% | 12.0% | 18.0% | 12% |
| Return to debtholders (%) | − | − | − | − |
| Return to shareholders (%) | 6.0% | 12.0% | 18.0% | 12% |
| Earnings per share | 0.60 | 1.20 | 1.80 | 1.20 |
These takeaways are as follows:
- Since we have assumed no taxes and no debt financing, the firm neither pays interest nor taxes. Consequently, in this scenario, net income corresponds to EBIT.
- This is also the total amount of money that is available for distribution to the providers of capital—in this case the shareholders (equity cash flow).
- Depending on the future state of the world, shareholders will therefore receive a payment of 300, 600, or 900, respectively. The average expected equity cash flow is 600.
- Given a current equity value of 5'000, as per our assumption, the shareholders' average expected return is 12%. This is the cost of equity:
Average expected return to shareholders = Cost of equity = \( \frac{\text{Equity cash flow}}{\text{Equity value}} = \frac{600}{5'000} \) = 12%.
- However, this return is not constant. In a negative outcome (recession), the shareholders' return can be as low as 6% [= 300/5'000] whereas in a positive outcome, it can be as high as 18% [= 900/5'000]. On average, as we have already seen, shareholders expect to earn a return of 12%.
- Finally, given 500 shares outstanding, the average expected earnings per share (EPS) is 1.2:
Average Earnings per share (EPS) = \( \frac{\text{Net income}}{\text{Number of shares}} = \frac{600}{500} \) = 1.2.
Assuming perpetual cash flows, we can now also replicate the valuation of the firm's equity. Using the equation from the previous page, the equity value is:
Equity value = \( \frac{\text{Equity cash flow}}{\text{Cost of equity}} = \frac{600}{0.12} \) = 5'000.
Since there are 500 shares outstanding, the theoretical stock price is 10 [= 5'000/500], which corresponds to the actual stock price. We can see this more direclty by valuing the stock using the per-share equation from the previous page:
Stock price = \( \frac{EPS}{\text{Cost of equity}} = \frac{1.2}{0.12} \) = 10.
Let us now investigate how this analysis changes if the firm decides to finance parts of its activities with debt. This is the topic of the next section.